Question

Sketch the graph of and identify the axis of symmetry


`y = - 4(x + 4) ^(2) + 4`
A. x=4

B.x=-16

C.x=16

D. x=-4

Answer 1

The axis of symmetry is found within the set of parenthesis with the x. If our h value of the vertex is -4, then the axis of symmetry is x = -4. D is that choice. Cannot graph it here, but your vertex is sitting at (-4, 4), it's an upside down parabola, and some other points on this graph are (-5, 0), (-3, 0), (-6, -12), (-2, -12). You could graph it using those points and the vertex without a problem, I'm sure.

Answer 2

Axis of symmetry is x = -4.

Explanation:

The given equation is
`y=-4(x+4)^2+4`

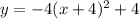
It represents a downward parabola because a = -4 <0

The vertex form of a parabola is

`y=a(x-h)^2+k`

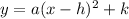
Where (h,k) is the vertex and x = h is the axis of symmetry.

Thus, the vertex of the given parabola is (-4,4) and the axis of symmetry is x = -4.

The vertex will be at (-4,4) and is the lowest point of the graph of the parabola.

The x-intercept are

`0=-4(x+4)^2+4\\\\-4(x+4)^2=-4\\\\(x+4)^2=1\\\\x+4=\pm1\\\\x=-1-4,1-4\\\\x=-5,-3`

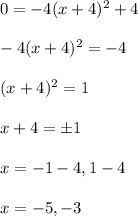
And the y-intercepts is

`y=-4(0+4)^2+4\\\\y=-4(16)+4\\\\y=-64+4\\\\y=-60`

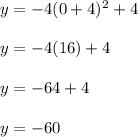
Thus, using these facts we can draw the graph which is shown below.

Axis of symmetry is x = -4.

D is the correct option.