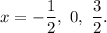Answer: The solutions of the given equation in increasing order are
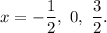
Step-by-step explanation: The given equation is

We are to solve the above equation and to list the solutions in increasing order.
We know that

So, from equation (i), we get
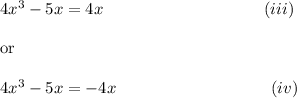
Solving equation (iii), we get
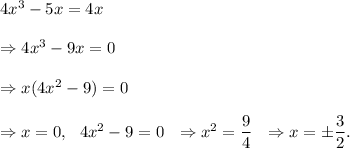
So, solutions of equation (i) are

And, solving equation (iv), we get
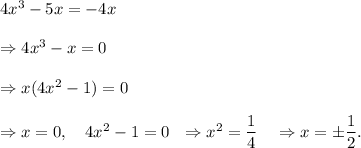
So, solutions of equation (ii) are
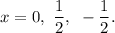
We can see that
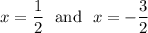 does not satisfy equation (i).
does not satisfy equation (i).
For
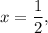 we have
we have

Similarly, for
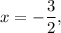 we have
we have
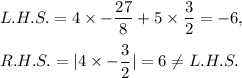
Thus, the solutions of the given equation in increasing order are