Question

There are 3 green balls, 5 red balls and 2 blue balls in a box. One ball is randomly drawn, replaced and then another ball is drawn.

What is the probability of getting a green ball than a blue ball?

Answers:
1/2
3/50
3/100
1/15

Answer 1

The probability of getting a green ball at the first drawn
p₁ =
`(green)/(total)`
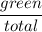
p₁ =
`(3)/(3+5+2)`
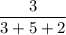
p₁ =
`(3)/(10)`

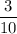
The green ball is replaced, that means the total ball is still 10.
The probability of getting a blue ball at the second drawn
p₂ =
`(blue)/(total)`
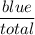
p₂ =
`(2)/(10)`
p₂ =
`(1)/(5)`

The probability of both event
p = p₁ × p₂
p =
`(3)/(10) * (1)/(5)`
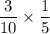
p =
`(3)/(50)`

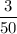The probability is 3/50