Question

Write a conditional statement that correctly describes each venn diagram in the space provided?

Answer 1

Remember that a conditional statement (p→q) is an if-then in which the first part, p, is the hypothesis and the second part, q, is the conclusion.

1. Here the set m∠A=40° is a subset of the set Acute Angles, so m∠A=40° is the hypothesis and Acute Angles is the conclusion.
In other words:
m∠A=40°→∠A is an Acute Angle
if m∠A=40°, then ∠A is an Acute Angle

We can conclude that the conditional statement that describes the Venn diagram is: if m∠A=40°, then ∠A is an Acute Angle

2. Just like before, the set
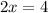`2x=4` is a subset of the set
`x=2`; therefore,
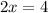`2x=4` is the hypothesis and
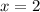`x=2` is the conclusion.
In other words:

`2x=4`→
`x=2`
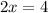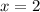
if
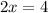`2x=4`, then
`x=2`

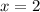
We can conclude that the conditional statement that describes the Venn diagram is: if
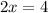`2x=4`, then
`x=2`