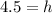Question

A sphere and the base of a cone have a radius of 3 inches. The volume of the sphere equals the volume of the cone. what is the height of the cone, in inches?

Answer 1

So the volume of a sphere is
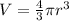
`V= (4)/(3) \pi r^3` , and the volume of a cone is
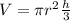
`V= \pi r^2 (h)/(3)` (h=height, r=radius). Knowing only the radius, we can solve for the volume of the sphere. (Also I'm going to be leaving answers in pi form.)


`V= (4)/(3) \pi 3^3`
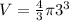
Solve the exponents to get
`V= (4)/(3) \pi 9`
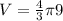
Multiply 4/3 and 9 to get
`V=13.5 \pi`


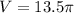
Now that we know the volume of the sphere, we can solve for the height of the cone since the volumes for both are the same.


`13.5 \pi = \pi 3^2 (h)/(3)`

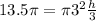
Solve the exponents to get
`13.5 \pi =9 \pi (h)/(3)`

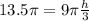
Divide
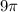
`9 \pi` on each side to get
`1.5= (h)/(3)`

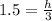
Multiply 3 on each side, and your answer should be
`4.5=h`