Answer: The required value of the limit is 18.
Step-by-step explanation: We are given to find the limit of the following function algebraically :
limit as x approaches nine of quantity x squared minus eighty one divided by quantity x minus nine.
We will be using the following factorization formula :
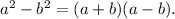
The limit can be calculated as follows :
![\ell\\\\\\=\lim_(x\rightarrow 81)(x^2-81)/(x-9)\\\\\\=\lim_(x\rightarrow 9)(x^2-9^2)/(x-9)\\\\\\=\lim_(x\rightarrow 9)((x+9)(x-9))/((x-9))\\\\=\lim_(x\rightarrow 9)(x+9)~~~~~~~~~~~~~~~~[\textup{since }x\rightarrow 9,~so~x\\eq 9]\\\\=9+9\\\\=18.](https://img.qammunity.org/2019/formulas/mathematics/high-school/xnjjgzh09069ipmz4z1n1p9h6g449qe40r.png)
Thus, the required value of the limit is 18.