Question

A mixture of KCl and KNO3

is 44.20% potassium by mass. The percent of KCl in the
mixture is closest to
a) 40%
b) 50%
c) 60%
d) 70%
e) 80%

Answer 1

The correct answer is option (a).

Step-by-step explanation:

Suppose we have total mass of the mixture be 100 g

let the mass of KCl be x

let the mass of
`KNO_3` be y

x + y = 100...(1)

Moles of KCl =
`(x)/(75.5 g/mol)`

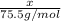
Moles of K in KCl will be =
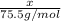`(x)/(75.5 g/mol)` (1 mol is present in 1 mole of molecule)

Moles of
`KNO_3=(y)/(102 g/mol)`

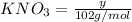
Moles of K in
`KNO_3` will be =
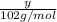`(y)/(102 g/mol)` (1 mol is present in 1 mole of molecule)

Percentage of potassium in the mixture = 44.20 %

In 100 g, the mass of potassium = 44.20 g

Moles of potassium =
`(44.20 g)/(40 g/mol)=1.105 mol`

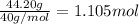
So,

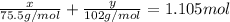`(x)/(75.5 g/mol)+(y)/(102 g/mol)=1.105 mol`..(2)

Solving equation (1) and (2):

we get ,y = 63.78 g, x=36.22 g

Percentage of KCl:

`(36.22 g)/(100g)* 100=36.22 g\%`

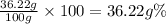
The percent of KCl in the mixture is closest to 40%.Hence ,option (a) is correct.

Answer 2

Answer is: The percent of KCl in the mixture is closest to a) 40%.

If we use 100 grams of mixture:
ω(K) = 44.20% ÷ 100% = 0.442.
m(K) = 0.442 · 100 g = 44.2 g.
n(K) = 44.2 g ÷ 39.1 g/mol.
n(K) = 1.13 mol.
n(KCl) + n(KNO₃) = n(K)
m(KCl) = x.
m(KNO₃) = y.
Two equtions:
1) x + y = 100 g.
2) m(KCl)/M(KCl) + m(KNO₃)/M(KNO₃) = 1.13 mol.
x/74.55 g/mol + y/101.1 g/mol = 1.13 mol.
From first equation find x = 100 - y and put in second equation:
(100 - y)/74.55 + y/101.1 = 1.13 /×101.1.
135.61 - 1.356y + y = 114.24.
0.356y = 22.37 g.
y = 62.83 g.
x = m(KCl) = 100 g - 62.83 g = 37.17 g.