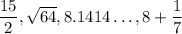Question

Order the set of numbers from least to greatest: square root 64, 8 and 1 over 7, 8.14 repeating 14, 15 over 2

A 15 over 2, square root 64, 8 and 1 over 7, 8.14 repeating 14
B 8 and 1 over 7, 8.14 repeating 14, square root 64, 15 over 2
C square root 64, 8 and 1 over 7, 8.14 repeating 14, 15 over 2
D 15 over 2, square root 64, 8.14 repeating 14, 8 and 1 over 7

PLEASE HELP

Answer 1

D.

Explanation:

15/2, √64, 8.14 repeating, 8 1/7

Answer 2

First, some rewriting:


`√(64)=8`


`8.1414\ldots=(806)/(99)`

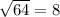
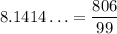
Why? If
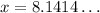
`x=8.1414\ldots`, then
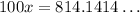
`100x=814.1414\ldots`, which means
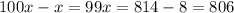
`100x-x=99x=814-8=806`, and so
`x=(806)/(99)`.


`\frac{15}2=7.5`

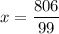
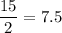
So from the four given numbers, it's obvious that
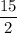
`\frac{15}2` is the smallest, followed by
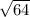
`√(64)`. So you just need to determine which of
`8.1414\ldots` and
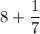
`8+\frac17` is smallest.

Note that


`8+\frac17=\frac{57}7`

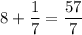
To easily compare this number to
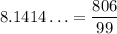
`8.1414\ldots=(806)/(99)`, we need to find a common denominator. The greatest common divisor of 7 and 99 is 1 (they are relatively prime), so
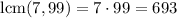
`\mathrm{lcm}(7,99)=7\cdot99=693`. So we write



`\frac{57}7\cdot(99)/(99)=(5643)/(693)`

`(806)/(99)\cdot\frac77=(5642)/(693)`

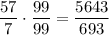
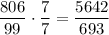
So we find that
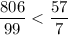
`(806)/(99)<\frac{57}7`, that is,
`8.1414\ldots<8+\frac17`.

So the proper ordering from least to greatest is


`\frac{15}2,√(64),8.1414\ldots,8+\frac17`