(a) By DeMoivre's theorem, we have
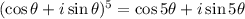
On the LHS, expanding yields
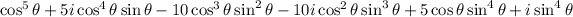
Matching up real and imaginary parts, we have for (i) and (ii),
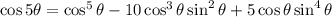
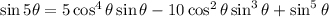
(b) By the definition of the tangent function,
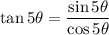

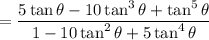

(c) Setting
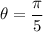
, we have
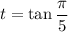
and
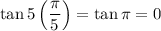
. So
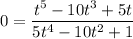
At the given value of

, the denominator is a non-zero number, so only the numerator can contribute to this reducing to 0.

Remember, this is saying that
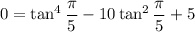
If we replace
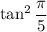
with a variable

, then the above means
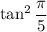
is a root to the quadratic equation,
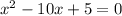
Also, if
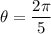
, then

and
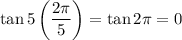
. So by a similar argument as above, we deduce that
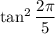
is also a root to the quadratic equation above.
(d) We know both roots to the quadratic above. The fundamental theorem of algebra lets us write
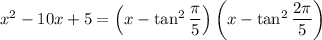
Expand the RHS and match up terms of the same power. In particular, the constant terms satisfy
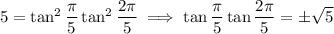
But
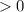
for all
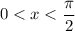
, as is the case for
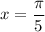
and
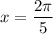
, so we choose the positive root.