Answer:
a) The building is 150 m tall
b) h(t)=-5(t+6)(t-5)
c) The stone hits the ground at 5 seconds.
Explanation:
Function Models
The path of a stone thrown down from a tall building is modeled by the function:
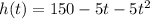
Where h is the height in meters and t is the time in seconds after the stone is thrown.
a) To find the height of the building, we set t=0 because it's the moment when the stone is thrown and has the same height as the building:
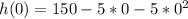

The building is 150 m tall
b) The function is
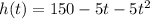
Factoring out -5:
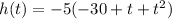
Rearranging:
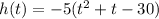
To factor the polynomial in parentheses, we must find two numbers whose product is -30 and sum is 1. We can also use the quadratic formula to find the roots and factor later.
These numbers are 6 and -5, thus the function is:
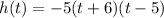
c) The stone hits the ground when h=0:
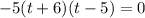
The equation has two solutions:
t = -6
t = 5
The only feasible solution is t = 5, thus the stone hits the ground at 5 seconds.