Question

Determine which of the following statements is true concerning the values described in column #1 and column #2. Column #1 Column #2 The x-coordinate of the vertex of the graph of y = −2x2 − 4x + 12 The x-coordinate of the vertex of the graph of y = x2 − 4x + 3

Answer 1

Hi,


1)

`y=-2x^2-4x+12=-2(x^2+2x+1)+2+12=-2(x+1)^2+14\\\\ Vertex=(-1,14) \ x-coordinate=-1\\`


2)

`y=x^2-4x+3=x^2-4x+4-1=(x-2)^2-1\\\\ Vertex=(2,-1) \ x-coordinate=2\\`

Answer 2

Answer with explanation:

→→The equation of curve 1 ,which is in the shape of parabola is ,when represented in general form

`y= -2x^2-4 x + 12\\\\ y=-2[x^2+2 x-6]\\\\ (y)/(-2)=(x+1)^2-6-1\\\\ (-y)/(2)+7=(x+1)^2`

So, Coordinate of vertex can be obtained by

→ x+1=0

x= -1

and,
`(-y)/(2)+7=0\\\\ y=14`

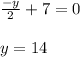
is equal to , (-1,14).

x-Coordinate of vertex of the function ,
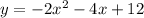`y=-2x^2-4 x + 12` is equal to -1.

→→→The equation of curve 2 ,which is in the shape of parabola is ,when represented in general form

`y= x^2-4 x + 3\\\\ y=(x-2)^2+3-4\\\\ y=(x-2)^2-1\\\\ y+1=(x-2)^2`

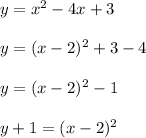
So, Coordinate of vertex can be obtained by

→ x-2=0

x= 2

and, →y+1=0

y= -1

is equal to , (2,-1).

x-Coordinate of vertex of the function ,
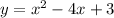`y=x^2-4 x + 3` is equal to 2.