Answer: The correct statements are
(B) Angle X is the largest angle.
(C) angle Z is greater than angle Y.
(E) XZ is the shortest side.
Step-by-step explanation: We are given a triangle XYZ, with the lengths of the sides as follows:
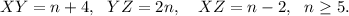
We have
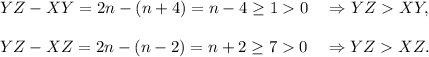
So, YZ is the longest side. Option (A) is incorrect.
Since YZ is the longest side, so the angle opposite to YZ will be the largest.
Therefore, ∠X is the largest angle. Option (B) is correct.
We have
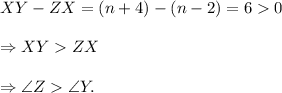
Option (C) is also correct.
The largest angle is ∠X, so its opposite side is YZ. Option (D) is incorrect.
Since XZ is smaller than XY and YZ, so XZ is the shortest side of the triangle XYZ. Option (E) is correct.
Thus, the correct statements are
(B) Angle X is the largest angle.
(C) angle Z is greater than angle Y.
(E) XZ is the shortest side.