Question

Which geometric series converges?

A. 1/2 + 1/4 + 1/8 + 1/16 + ...

B. 1/2 + 1 + 2 + 4 + ...

C. 1/2 + 3/2+ 9/2+ 27/2+...

D. 1/2 + 3 + 18 + 108 + ...

Answer 1

The answer is A. because

A.

`0.5 > 0.25 > 0.125 > 0.06125`
B.

`0.5 < 1 < 2 < 4`
C.

`0.5 < 1.5 < 4.5 < 13.5`
D.

`0.5 < 3 < 8 < 108`

Answer 2

Option: A is the correct answer.

A)
`(1)/(2)+(1)/(4)+(1)/(8)+(1)/(16)+.........`

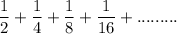
Explanation:

We know that a geometric series of the type:

`a_1+a_2+a_3+a_4+...`

where
`a_1=a\\\\a_2=ar\\\\a_3=ar^2\\.\\.\\.\\.\\.\\.`

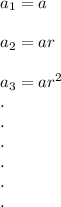
converges if r<1

Hence, we will check in each of the given options for which r<1

B)

`(1)/(2)+1+2+4+...`

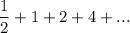
From this series we observe that the common ratio i.e. r=2

Since,

`a_1=(1)/(2)\\\\a_2=(1)/(2)* 2\\\\\\a_2=1\\\\a_3=1* 2=2\\\\a_4=2* 2=4`

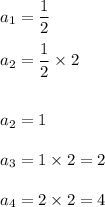
and so on.

Hence, the series is not convergent.

Hence, option: B is incorrect.

C)

`(1)/(2)+(3)/(2)+(9)/(2)+(27)/(2)+....`

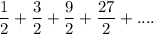
From this series we observe that the common ratio i.e. r=3>1

Hence, the series diverges.

Hence, option: C is false.

D)

`(1)/(2)+3+18+108+....`

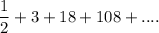
In this geometric series trhe common ratio is: 6>1

Hence, the series does not converge.

Hence, option D is incorrect.

A)

`(1)/(2)+(1)/(4)+(1)/(8)+(1)/(16)+.........`

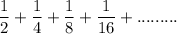
Here we have:

common ratio i.e. r=1/2<1

Hence, the geometric series converge and the sum is given by:

`S=(a)/(1-r)\\\\\\S=((1)/(2))/(1-(1)/(2))\\\\\\S=((1)/(2))/((1)/(2))=1`

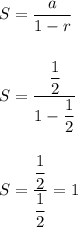
Hence, option: A is correct.