Question

The function F(x)=log0.75^x is decreasing. True or false

Answer 1

Correct Answer It's Increasing

Answer 2

We're given the following function:

`f(x)=log(.75^x)=log[ ((3)/(4)) ^(x)]=log( (3^x)/(4^x))`

In order to see if the function is decreasing we'll take its derivative. If
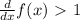
`(d)/(dx)f(x)\ \textgreater \ 1` the function is increasing, if
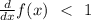
`(d)/(dx)f(x)\ \ \textless \ \ 1` the function is decreasing.

We take the derivate:

`(d)/(dx)[log( (3^x)/(4^x))]= (4^x)/(3^x)[ (d)/(dx) ((3^x)/(4^x))]=(4^x)/(3^x) (4^x[ (d)/(dx) (3^x)]-3^x[ (d)/(dx) (4^x)])/( 4^2^x)=`

`(4^x)/(3^x) (4^x[3^xlog(3)]-3^x[4^x[4^xlog(4)])/( 4^2^x)=log(3)-log(4)\ \textless \ 0`

Which implies the function is decreasing.

Another way to answer the problem (although less insightful) you can take any two real numbers
`k` and
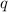
`q` such that
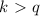
`k\ \textgreater \ q`, then if
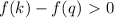
`f(k)-f(q)\ \textgreater \ 0` the function is increasing and if
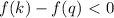
`f(k)-f(q)\ \textless \ 0` the function is decreasing. You can verify the function is decreasing with any two numbers in the function's domain.