Question

A sheet of paper is cut into 2 ​same-size parts. Each of the parts is then cut into 2 ​same-size parts and so on.

a. After the 7th ​cut, how many of the smallest pieces of paper are​ there?
b. After the nth​ cut, how many of the smallest pieces of paper are​ there?

Answer 1

the 7th cut is 118 and the 9th cut is 472

Answer 2

a) 128 b) 512

Explanation:

The key to solve this, is to think of it as a Geometric Sequence. Since, there is a constant ratio (q), each time the sheet of paper is cut, it is divided into two pieces or 1/2.

`{(1)/(2),(1)/(4),(1)/(8),(1)/(16),(1)/(32),(1)/(64),(1)/(128),(1)/(256),(1)/(512)...`

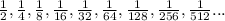
If after the 1st cut there are two pieces i.e. 1/2

a) After the 7th ​cut, how many of the smallest pieces of paper are​ there?

You can either count the sequence above: 1/128 then 128 pieces or apply the recursive formula for any Term of a Geometric Sequence;

`a_(n)=a_(1)* q^(n-1)\\ a_(7)=(1)/(2)*((1)/(2))^(6) \\ a_(7)=(1)/(128)`

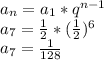
The Denominator indicates the smallest pieces of paper: 128

b) After the 9th​ cut, how many of the smallest pieces of paper are​ there?

Similarly to the item a: 1/512 then 512

`a_(n)=a_(1)* q^(n-1)\\ a_(9)=(1)/(2)*((1)/(2))^(8) \\ a_(9)=(1)/(512)`

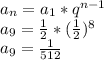
The Denominator indicates the smallest pieces of paper after the Ninth cut: 512