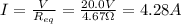First of all, let's calculate the equivalent resistance of the three resistors in parallel:
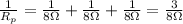

The 2.0-ohm resistance is in series with these resistors, so the equivalent resistance of the circuit is
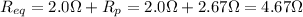
And the current in the circuit can now be calculated by using Ohm's law: