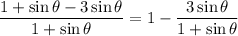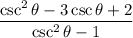
Identity:

So we can rewrite the denominator to get
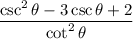
Multiply numerator and denominator by
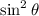
. Several terms will cancel since

. Also,
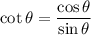
. We get
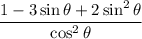
Factorize the numerator, and write
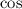
in terms of
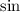
in the denominator to factorize it further to get
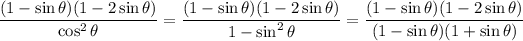
The
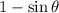
factors cancel, leaving you with
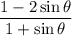
which you could simplify a bit further by writing