Question

One x-intercept for a parabola is at the point (-3,0). Use the factor method to find the other x-intercept for the parabola defined by this equation:

y= -x^2 - 5x - 6

separate the values with a comma.

Answer 1

A trinomial is given by the following formula:


`ax^(2) + bx + c`

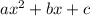
Identify a, b, and c in this trinomial:


`a = -1, b = -5, c = -6`

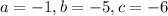
We'll pretend that all the numbers are positive.

Find all the factors of c:

Factors of 6: {1,2,3,6}

The numbers that multiply to 6 must also add up to b.

1 + 6 = 7
2 + 3 = 5

2 and 3 add up to 5. These numbers will be used for factoring:


`(x+2)(x+3)`

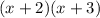
Because all of the numbers are negative, we have to add a negative sign in front of our factors:


`-(x+2)(x+3)`

To find the other x-intercept, one of the values must be set to 0.


`x + 2 = 0`

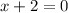
Subtract both sides by 2.


`x = -2`

The other x-intercept for this trinomial is x = -2.

Answer 2

An x-intercept is when y = 0

Set Y to zero in the given formula and then solve for X

y = -x^2 -5x -6

y = 0

0 = -x^2-5x-6

Factor the polynomial:

-(x+2) (x+3) = 0

x +2 = 0
x =-2

x+3 = 0
x = -3 ( Already given in the problem)

The other x intercept would be (-2,0)