Answer:
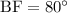
Explanation:
We have been given a circle D. Secant BE and CF intersect at point A inside D. We are asked to find the measure of arc BF.
We know that when two secants intersect inside a circle, then the measure of angle formed is half the sum of intercepting arcs.
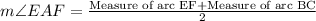
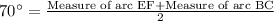
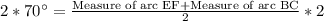
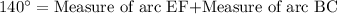
We know that degree measure of circumference of circle is 360 degrees, so we can set an equation as:

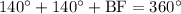
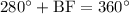
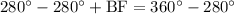
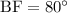
Therefore, the measure of arc BF is 80 degrees.