Question

What is the area of △ABC ?

Enter your answer, as a decimal, in the box.

Round only your final answer to the nearest tenth.


units²

Answer 1

59.5 units

Explanation:

took the quiz

Answer 2

`59.5\text{ units}^2`

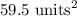
Explanation:

We have been an image of triangle ABC. We are asked to find the area of our given triangle.

We will use trigonometric formula to find area of triangle, when two sides and one included of a triangle is given:

`\text{Area of triangle}=(1)/(2)(ab)* \text{sin}(C)`, where, a and b represents lengths of sides, and C represents included angle.

Upon substituting our given values in above formula, we will get

`\text{Area of triangle ABC}=(1)/(2)(13\cdot 13)* \text{sin}(44.8^(\circ))`

`\text{Area of triangle ABC}=(1)/(2)(169)* 0.704634209964`

`\text{Area of triangle ABC}=84.5* 0.704634209964`

`\text{Area of triangle ABC}=59.541590741958\approx 59.5`

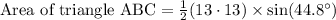
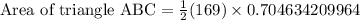
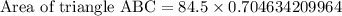
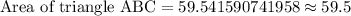
Therefore, the area of triangle ABC is 59.5 square units.