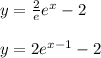The parent exponential function is:
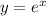
The Horizontal asymptote of parent exponential function is y =0. For the function to have asymptote y=-2, it must be shifted 2 units down. So resulting graph will be:
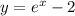
The function is increasing from left to right.
The above function does not have x-intercept at (1,0). For the function to have an x-intercept at (1,) it must be multiplied with some co-efficient as shown below:
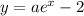
For x=1, y=0. So we can write:
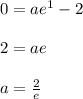
So, the exponential function satisfying the given conditions will be: