Answer:
The statements which are true for the functions f(x) and g(x) are:
B) The average rate of change of f(x) is less than the average rate of change of g(x).
E) The average rate of change of f(x) is -0.1, and the average rate of change of g(x) is -0.25.
Explanation:
The average rate of change of a function is the ratio of the difference in x-value to the difference in y-value.
i.e. for a function f(x) the average rate of change of the function f(x) over the interval [a,b] is given by:
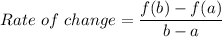
- The table of the function f(x) is given by:
x f(x)
3 1.33
4 1
5 0.8
6 0.66
10 0.4
Hence, the average rate of the function over [4,10] is:
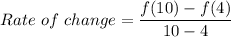
i.e.
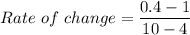
i.e.
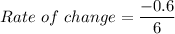
i.e.
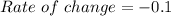
- By the graph of the function g(x) we observe that:
g(4)=2.5 and g(10)=1
Hence, the average rate of change of the function g(x) over the interval [4,10] is:

i.e.
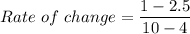
i.e.
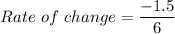
i.e.
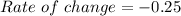
Since the modulus of the average rate of change for the function g(x) is greater than the function f(x)
Hence, g(x) has a greater average rate of change than f(x).
( Since, 2.5>0.1)