Question

An electron begins at rest, and then is accelerated by a uniform electric field of 800 n/c that extends over a distance of 4 cm. find the speed of the electron after it leaves the region of uniform electric field. the fundamental charge is 1.602 × 10−19 c and the mass of the electron is 9.109 × 10−31 kg. answer in units of m/s.

Answer 1

The force experienced by the electron due to the electric field is given by:

`F=qE=(1.6 \cdot 10^(-19)C)(800 N/C)=1.28 \cdot 10^(-16)N`

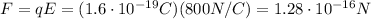
The work done by the field over a distance of d=4 cm=0.04 m is equal to the energy acquired by the electron during its motion:

`W=\Delta E= Fd=(1.28 \cdot 10^(-16)N)(0.04 m)=5.12 \cdot 10^(-18)J`

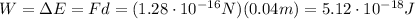
Since the electron starts from rest, its initial kinetic energy is zero, so this energy acquired is equal to the final kinetic energy of the electron:

`K=5.12 \cdot 10^(-18)J`
And from the formula

`K= (1)/(2)mv^2`
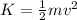
we can find the speed of the electron:

`v= \sqrt{ (2K)/(m) } = \sqrt{ (2(5.12 \cdot 10^(-18) J))/(9.11 \cdot 10^(-31) kg) }=3.35 \cdot 10^6 m/s`