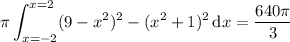Question

find the volume of the solid that is generated by rotating around the indicated axis: y=x^2, y=8-x^2 the line y= -1

Answer 1

Another method is to use Pappus's theorem, which states that if a region with area A is rotated about a line L, the volume of revolution generated is equal to
V=2(pi)RA, where R=distance of the centroid of region from the axis of rotation.

Here,

`A=\int_(-2)^(2)(8-x^2-x^2)dx=64/3`
Centroid (from symmetry) passes through y=4.
Volume = 2 π (4+1)(64/3) = 640 π /3 (approximately =670.2 ) units ³

Answer 2

Use the washer method. Refer to the attached image below, which shows one such washer used to approximate the volume.

The volume of this kind of washer is
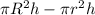
`\pi R^2h-\pi r^2h`, where
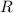
`R` is the radius of the larger cylinder and
`r` is the radius of the smaller cylinder. The height
`h` is the same for both and is given by
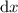
`\mathrm dx`, an infinitesimal change in
`x`. The radius of the larger cylinder is
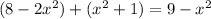
`(8-2x^2)+(x^2+1)=9-x^2`, while the radius of the smaller cylinder is just
`x^2+1`.

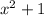
The two parabolas intersect at
`x=\pm2`, so the volume of this solid is given by



`\displaystyle\pi\int_(x=-2)^(x=2)(9-x^2)^2-(x^2+1)^2\,\mathrm dx=\frac{640\pi}3`