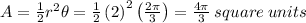Refer to the figure.
We are looking for the area of the sector of a circle as shown in the figure shaded with green color.
The area of a sector of a circle can be calculated using the formula
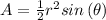
where r=radius, and θ=central angle (in radians)
The central angle of the given sector is just one-third of a full circle (2π). That is
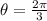
Now, to solve for the radius of the circle, we can use the formula
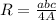
where R is the radius of the circumscribed circle; a,b, and c are the sides of the triangle; and A is the area of the triangle.
The area of the equilateral triangle can be solved using the formula
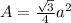
. That is

Now, we substitute this area in the formula to solve for the radius of the circle.
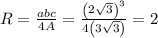
Finally, we can solve for the area of the sector by substituting the values of the angle θ, and the radius.