Question

The radius of circle O is 24,and OC = 11. What is the length of AB?

Answer 1

The answer is A.

42.7

Answer 2

Answer: The correct option is (A) 42.7 units.

Step-by-step explanation: Given that the radius of the circle center at O is 24 units and OC is 11 units.

We are to find the length of chord AB.

We have,

in the right-angled triangle OCB (m∠OCB = 90°),

OB = 24 units and OC = 11 units.

Using Pythagoras theorem in ΔOCB, we have

`OB^2=OC^2+BC^2\\\\\Rightarrow BC=√(OB^2-OC^2)\\\\\Rightarrow BC=√(24^2-11^2)\\\\\Rightarrow BC=√(576-121)\\\\\Rightarrow BC=√(455)\\\\\Rightarrow BC=21.33.`

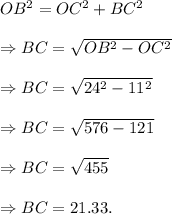
Since OC is perpendicular to chord AB, so AC = BC.

Therefore, we get

`AB=AC+BC=2* BC=2* 21.33=42.66=42.7.`

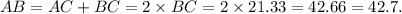
Thus, the required length of AB is 42.7 units.

Option (A) is CORRECT.