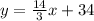Answer:
Slope - intercept form
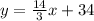
Explanation:
Explanation:-
Given points are ( -9 ,-8 ) and (-6,6)
slope of given two points
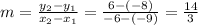
The equation of the straight line passing through the points and having slope

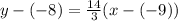
3 y + 2 4 = 14 x + 126
3 y = 14 x + 126 - 24
3 y = 14 x + 102
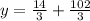
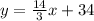
Slope - intercept form y= mx +C
Slope - intercept form