Answer:
C. Compressed horizontally by a factor of 1/3, horizontal shift right 2, vertical shift up 3
Explanation:
If a function f(x) is transformed and make f(kx),
f(x) is compressed horizontally by the factor 1/k if k > 1
f(x) is stretched horizontally by the factor 1/k if 0 < k < 1
Also, if f(x) is transformed and make f(x+a),
f(x) is shifted right if a < 0
f(x) is shifted left if a > 0,
Now, if f(x) gives f(x) + k after transformation,
f(x) is shifted vertically k unit up if k > 0,
f(x) is shifted vertically k unit down if k < 0,
Here, the parent function,
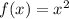
transformed function,
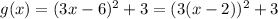
Thus, by the above explanation,
f(x) is compressed horizontally by a factor of 1/3, horizontal shift right 2, vertical shift up 3.
Option C is correct.