We know that the exponential growth of the Bacteria's population is model by the function
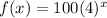
, so to find the number of bacteria after 6 hours, we just need to evaluate our function at
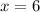
. In other words, we are going to replace

with 6 in our function:
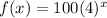
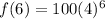

We can conclude that the we should use the equation:
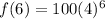
to find the number of bacteria in the colony after 6 hours. Evaluating the function we get that the number is
409,600.