Question

In ΔАВС, m∠ACB = 90°,
CD
⊥
AB
m∠ACD = 30°, and AC = 6 cm. Find BD

Answer 1

It is 9 because you have a 30,60,90 triangle, and then you can use pythagorean theorem.

Answer 2

Given is a Right triangle ΔACB with angle ∠ACB = 90 degrees.

Given that CD⊥AB, it means we have two Right triangles ΔCDA and ΔCDB.

Given angle ∠ACD = 30 degrees, it means ∠A = 60° and ∠B = 30°

Given side AC = 6 centimeters.

It says to find BD = ?

In Right triangle ΔCDA; CD is adjacent, AC is hypotenuse, ∠ACD = 30°

`Cos(\angle ACD)=(adjacent)/(hypotenuse) \\\\Cos(30^o)=(CD)/(AC) \\\\(√(3))/(2) =(CD)/(6) \\\\CD = 3√(3) \;cm`

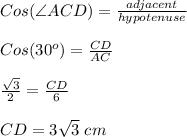
In Right triangle ΔCDB; CD is opposite, BD is adjacent, ∠B = 30°

`Tan(\angle B) = (opposite)/(adjacent) \\\\Tan(30^o) = (CD)/(BD) \\\\(1)/(√(3)) = (3√(3))/(BD) \\\\Cross \;\;multiplying \\\\BD = √(3) * 3√(3) \\\\BD = 9 \;cm`

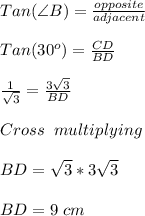
Hence, final answer is BD = 9 centimeters.