Question

Juan put three square tiles with sides 8 centimeters, 10 centimeters, and x centimeters together so that they form a right triangle. Which statement is true about the area A of the smallest tile?

Answer 1

since the area of a square is equal to the square of one of its side's length, then the area should be equivalent to
`x^(2)`.

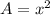
`A = x^(2)` ---> equation (1)
By using pythagoras rule which states that the
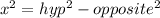
`x^(2) = hyp^2 - opposite^2`---> equation (2)
where the opposite side's length is 8 and the hypotenuse side's length is 10
by substituting by the values in equation (2) therefore,

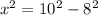
`x^(2) = 10^(2) - 8^(2)` substitute this value in equation (1) then

`A = x^(2) = 10^(2) -8^(2)`
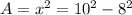
where A is the area of the square whose side is x

Answer 2

The smallest triangle is not going to be determined by the a plus sign between the largest square and one of the small ones. That eliminates the two top answers.

A right triangle has the pythagorean equation associated with it.

That's a^2 + b^2 = c^2

Both the top right answer and the bottom left answer are eliminated because they are not of this form.

The largest side is c so to find x you need to subtract 8^2 from 10^2

10^2 - 8^2 is the answer <<<<<