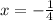Answer:
The x-coordinate of the intersection of the lines is
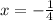
Explanation:
First you need to know the equation of the line that passes through the points (0,2) and (1,1).
The equation of the line in slope intercept form is y=m*x + b
By having two points, you can use them to find the slope m using the expression:
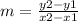
In this case (x1,y1)= (0,2) and (x2,y2)= (1,1). Replacing:
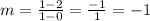
Now you can replace in the values of m, and the values of a point x and y into the equation y = mx + b to find the value of b.
1=(-1)*1 +b
So: 1=-1 +b
b=1+1
b=2
Then you have: y=-1*x +2
So you have the following system of equations:

Equating both equations you have:
-1*x+2= 3*x+3
Solving:
-1*x-3*x= 3-2
-4*x= 1
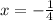
The x-coordinate of the intersection of the lines is