Question

Which of the following will induce a reduced emf in the secondary coil of a generator?

fewer number of loops in the secondary coil.
greater number of loops in the primary coil.
larger area in the secondary coil.
constant potential in the primary coil.

Answer 1

fewer number of loops in the secondary coil.

Step-by-step explanation:

The transformer equation states that:

`(V_p)/(N_p)=(V_s)/(N_s)`

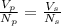
where

Vp is the voltage in the primary coil

Np is the number of loops in the primary coil

Vs is the emf induced in the secondary coil

Ns is the number of loops in the secondary coil

Solving the expression for Vs,

`V_s = (N_s)/(N_p)V_p`

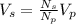
Therefore, we see that in order to reduce the emf in the secondary coil, we can reduce Ns, the number of loops in the secondary coil. So the correct option is

fewer number of loops in the secondary coil.