Answer:
The correct option is C.
Explanation:
Root Of Complex Numbers
If a complex number is expressed in polar form as
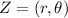
Then the cubic roots of Z are
![\displaystyle Z_1=\left(\sqrt[3]{r},(\theta)/(3)\right)](https://img.qammunity.org/2020/formulas/mathematics/middle-school/zw96jak3nx3icj6powziudyid7x1bi3hdn.png)
![\displaystyle Z_2=\left(\sqrt[3]{r},(\theta)/(3)+120^o\right)](https://img.qammunity.org/2020/formulas/mathematics/middle-school/7csndkkiy6qwn93av5ytvsa12pckco5xoa.png)
![\displaystyle Z_3=\left(\sqrt[3]{r},(\theta)/(3)+240^o\right)](https://img.qammunity.org/2020/formulas/mathematics/middle-school/rfaizg3k6k2x9cjeccq8d9ercnlrel4bdn.png)
We are given the complex number in rectangular components
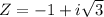
Converting to polar form
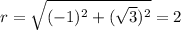
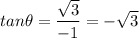
It's located in the second quadrant, so
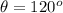
The number if polar form is
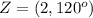
Its cubic roots are
![\displaystyle Z_1=\left(\sqrt[3]{2},(120^o)/(3)\right)=\left(\sqrt[3]{2},40^o\right)](https://img.qammunity.org/2020/formulas/mathematics/middle-school/s6fnbb53wkh992hkwzkm5m71gp68jdq2hx.png)
![\displaystyle Z_2=\left(\sqrt[3]{2},40^o+120^o\right)=\left(\sqrt[3]{2},160^o\right)](https://img.qammunity.org/2020/formulas/mathematics/middle-school/ts9oe038svza8oow3ajmjmd0x9ju0lzhcd.png)
![\displaystyle Z_3=\left(\sqrt[3]{2},40^o+240^o\right)=\left(\sqrt[3]{2},280^o\right)](https://img.qammunity.org/2020/formulas/mathematics/middle-school/mwjzfhjley3h4lfnq71hdrhmvw0z1678ow.png)
Converting the first solution to rectangular coordinates
![z_1=\sqrt[3]{2}(\ cos40^o+i\ sin40^o)](https://img.qammunity.org/2020/formulas/mathematics/middle-school/mposyn789nqtyur2ntstxso8lchm59np8u.png)
The correct option is C.