Question

A manufacturer wants to make open tin boxes from pieces of tin with dimensions 8 in. by 15 in. by cutting equal squares from the four corners and turning up the sides. Find the side of the square cutout that gives the box the largest possible volume.

0 in.
6 in.
52788.gif
52789.gif

Answer 1

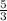`(5)/(3)` in

Explanation:

Let x be the side of square.

Length of box=8-2x

Width of box=15-2x

Height of box=x

Volume of box=
`L* B* H`

Substitute the values then we get

Volume of box=V(x)=
`(8-2x)(15-2x)x=(15x-2x^2)(8-2x)`

`V(x)=120x-30x^2-16x^2+4x^3`

`V(x)=4x^3-46x^2+120x`

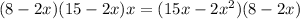
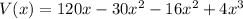
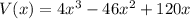
Differentiate w.r.t x

`V'(x)=12x^2-92x+120`

`V'(x)=0`

`12x^2-92x+120=0`

`3x^2-23x+30=0`

`3x^2-18x-5x+30=0`

`3x(x-6)-5(x-6)=0`

`(x-6)(3x-5)=0`

`x-6=0\implies x=6`

`3x-5=0\implies x=(5)/(3)`

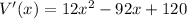
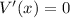
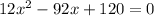
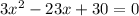
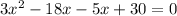
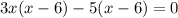
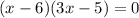
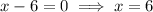
Again differentiate w.r.t x

`V''(x)=24x-92`

Substitute x=6

`V''(6)=24(6)-92=52>0`

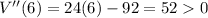
Substitute x=5/3

`V''(5/3)=24(5/3)-92=-52<0`

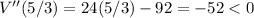
Hence, the volume is maximum at x=
`(5)/(3)`

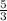
Therefore, the side of the square ,
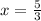`x=(5)/(3)` in cutout that gives the box the largest possible volume.