Question

In the function y+2=1/3(x-4)^2

, what effect does the number 4 have on
the graph, as compared to the graph of y=x"?

Answer 1

It shifts the graph up 4 units is the right answer, not to the right. I got that wrong.

Explanation:

Answer 2

The negative 4 translates the original function 4 units horizontally to the right

Explanation:

The transformed graph is
`y+2=(1)/(3)(x-4)^2`

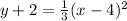
and the parent function is
`y=x^2`

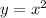
We need to find what effect does the "4" in the transformed function have with respect to the parent function. Lets look at a general form of a transformed quadratic function and see what each variable means:

`y+b=a(x-c)^2`

This is the general form.

• +b means the graph is vertically translated b units down
• -b would have meant the graph would have been vertically translated b units up
• a transforms the parent by vertically stretching or compressing
• -c means the parent is shifted c units right
• +c would have meant the parent will be shifted c units left

In our transformed graph, we have a "-4", this means, according to the rules above, that:

the parent function is shifted 4 units to the right