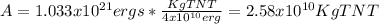Answer:
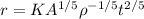
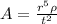
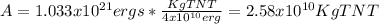
Step-by-step explanation:
Notation
In order to do the dimensional analysis we need to take in count that we need to conditions:
a) The energy A is released in a small place
b) The shock follows a spherical pattern
We can assume that the size of the explosion r is a function of the time t, and depends of A (energy), the time (t) and the density of the air is constant
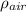 .
.
And now we can solve the dimensional problem. We assume that L is for the distance T for the time and M for the mass.
[r]=L with r representing the radius
[A]=
 A represent the energy and is defined as the mass times the velocity square, and the velocity is defined as
A represent the energy and is defined as the mass times the velocity square, and the velocity is defined as
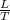
[t]=T represent the time
![[\rho]=(M)/(L^3)](https://img.qammunity.org/2020/formulas/engineering/college/crwvx6h33w4n41db5fnjovaoq4lnrt0p75.png) represent the density.
represent the density.
Solution to the problem
And if we analyze the function for r we got this:
![[r]=L=[A]^x [\rho]^y [t]^z](https://img.qammunity.org/2020/formulas/engineering/college/2ixg1powj6zazqm8v100qx8v16yao309pd.png)
And if we replpace the formulas for each on we got:
![[r]=L =((ML^2)/(T^2))^x ((M)/(L^3))^y (T)^z](https://img.qammunity.org/2020/formulas/engineering/college/xu8sewxcvmt8jc71ib5eorfuicf72r5cgt.png)
And using algebra properties we can express this like that:
![[r]=L=M^(x+y) L^(2x-3y) T^(-2x+z)](https://img.qammunity.org/2020/formulas/engineering/college/djhfuc4a9na5rp3y4h3m2uugusb2vdy0x1.png)
And on this case we can use the exponents to solve the values of x, y and z. We have the following system.
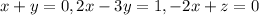
We can solve for x like this x=-y and replacing into quation 2 we got:

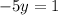
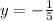
And then we can solve for x and we got:
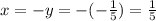
And if we solve for z we got:

And now we can express the radius in terms of the dimensional analysis like this:
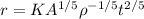
And K represent a constant in order to make the porportional relation and equality.
The problem says that we can assume the constant K=1.
And if we solve for the energy we got:
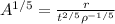
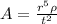
And now we can replace the values given. On this case t =0.025 s, the radius r =140 m, and the density is a constant assumed
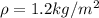 , and replacing we got:
, and replacing we got:
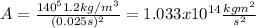
And we can convert this into ergs we got:
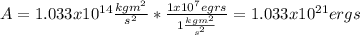
And then we know that 1 g of TNT have
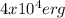
And we got: