Question

A. Evaluate ∫20 tan 2x sec^2 2x dx using the substitution u = tan 2x.

b. Evaluate ∫20 tan 2x sec^2 2x dx using the substitution u = sec 2x.
c. Reconcile the results in parts​ (a) and​ (b).

Answer 1

The integral is equal to
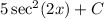`5\sec^2(2x)+C` for an arbitrary constant C.

Explanation:

a) If
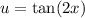`u=\tan(2x)` then
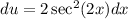`du=2\sec^2(2x)dx` so the integral becomes
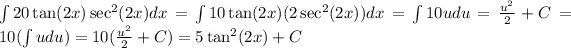`\int 20\tan(2x)\sec^2(2x)dx=\int 10\tan(2x) (2\sec^2(2x))dx=\int 10udu=(u^2)/(2)+C=10(\int udu)=10((u^2)/(2)+C)=5\tan^2(2x)+C`. (the constant of integration is actually 5C, but this doesn't affect the result when taking derivatives, so we still denote it by C)

b) In this case
`u=\sec(2x)` hence
`du=2\tan(2x)\sec(2x)dx`. We rewrite the integral as
`\int 20\tan(2x)\sec^2(2x)dx=\int 10\sec(2x) (2\tan(2x)\sec(2x))dx=\int 10udu=5(u^2)/(2)+C=5\sec^2(2x)+C`.

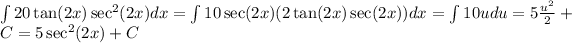c) We use the trigonometric identity
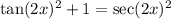`\tan(2x)^2+1=\sec(2x)^2` is part b). The value of the integral is
`5\sec^2(2x)+C=5(\tan^2(2x)+1)+C=5\tan^2(2x)+5+C=5\tan^2(2x)+C`. which coincides with part a)

Note that we just replaced 5+C by C. This is because we are asked for an indefinite integral. Each value of C defines a unique antiderivative, but we are not interested in specific values of C as this integral is the family of all antiderivatives. Part a) and b) don't coincide for specific values of C (they would if we were working with a definite integral), but they do represent the same family of functions.