Question

A boat whose speed is 15 km/hour in still water goes 36 km with stream and 24 km against the stream in a total of 4 hours. Find the speed of the stream.

Answer 1

`v_s=0\ km/h`

`v_s=3\ km/h`

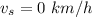
Step-by-step explanation:

Relative Speed

A boat traveling in still waters has a speed v_b. If now an opposite stream appears, then the apparent speed of the boat will be less than before because the relative speed is the subtraction of both speeds. Thus we say

`v_(t1)=v_b-v_s`

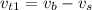
Conversely, if the stream goes with the boat, both speeds are added.

`v_(t2)=v_b+v_s`

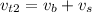
where
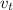`v_t` it the total or real speed of the boat respect to the ground,
`v_b` is the speed of the boat in still water, and
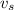`v_s` is the speed of the stream.

The boat goes 36 km with stream. The time it took for doing so is:

`\displaystyle t_1=(36)/(v_b+v_s)`

The boat goes 24 km against the stream in

`\displaystyle t_2=(24)/(v_b-v_s)`

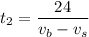
The problem states that

`t_1+t_2=4\ hours`

`\displaystyle (36)/(v_b+v_s)+(24)/(v_b-v_s)=4`

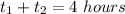
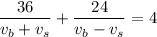
Knowing
`v_b=15`

`\displaystyle (36)/(15+v_s)+(24)/(15-v_s)=4`

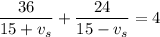
Operating

`\displaystyle 36(15-v_s)+24(15+v_s)=4(15+v_s)(15-v_s)`

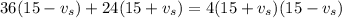
Rearranging and simplifying

`225-3v_s=225-v_s^2`

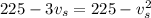
Factoring

`v_s(v_s-3)=0)`

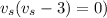
We get

`v_s=0,\ v_s=3`

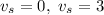
Both solutions are possible, i.e.

1. The stream does not exist and the boat travels the total distance of 60 Km in 4 hours

2. The stream has a speed of 3 Km/h and the boat travels 36 km in 2 hours and 24 km in 2 hours