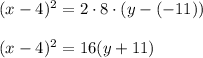Question

Derive the equation of the parabola with a focus at (4, −7) and a directrix of y = −15. Put the equation in standard form.

Answer 1

Answer: f(x)=1/16x^2-1/2x-10

Step-by-step explanation: I took the test and this is correct :)

Answer 2

`(x-4)^2=16(y+11)`

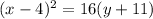
Explanation:

The directrix is horizontal line and focus is above the directrix, so the equation of the parabola will be in the form

`(x-x_0)^2=2p(y-y_0),`

where
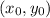`(x_0,y_0)` are the coordinates of the vertex.

The distance between the focus and the directrix is
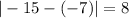`|-15-(-7)|=8` units, hence
`p=8.`

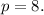
The vertex of the parabola is the point lying halfway from the focus to the directric on vertical line (parabola's axes of symmetry) x = 4, so its coordinates are (4,-11).

Therefore, the equation of parabola is

`(x-4)^2=2\cdot 8\cdot (y-(-11))\\ \\(x-4)^2=16(y+11)`