Question

An energy plant produces an output potential of 1500 kV and serves a city 143 km away. A high-voltage transmission line carries 500 A to the city. The effective resistance of a transmission line [wire(s)] is 2.4 Ω/km times the distance from the plant to the city. Consider the money lost by the transmission line heating the atmosphere each hour. Assume the energy plant produces the same amount of power; however, the output electric potential of the energy plant is 20 % greater. How much money per hour is saved by increasing the electric potential of the power plant? Answer in units of dollars/hr.

Answer 1

2123.55 $/hr

Step-by-step explanation:

Given parameters are:

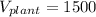`V_(plant) = 1500` KV

L = 143 km

I = 500 A

`\rho = 2.4`
`\Omega / km`

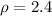
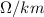
So, we will find the voltage potential provided for the city as:

`V_(wire) =IR = I\rho L = 1500*2.4*143 = 514.8` kV

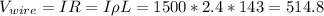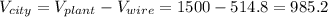`V_(city) = V_(plant)- V_(wire) = 1500-514.8 = 985.2` kV

Then, we will find dissipated power because of the resistive loss on the transmission line as:

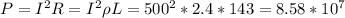`P = I^2R = I^2\rho L=500^2*2.4*143 = 8.58*10^7` W

Since the charge of plant is not given for electric energy, let's assume it randomly as
`x =  (\dollar 0.081)/(kW.hr)`

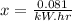
Then, we will find the price of energy transmitted to the city as:

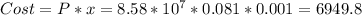`Cost = P * x = 8.58*10^7 * 0.081 * 0.001 = 6949.8` $/hr

To calculate money per hour saved by increasing the electric potential of the power plant:

Finally,

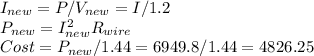`I_(new) = P/V_(new) = I/1.2\\P_(new) = I_(new)^2R_(wire)\\Cost = P_(new)/1.44=6949.8/1.44 = 4826.25` $/hr

The amount of money saved per hour =
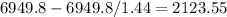`6949.8 - 6949.8/1.44 = 2123.55` $/hr

Note: For different value of the price of energy, it just can be substituted in the equations above, and proper result can be found accordingly.