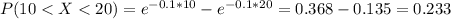Answer:
a)
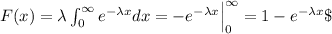
b)
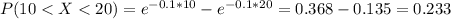
Step-by-step explanation:
Previous concepts
The cumulative distribution function (CDF) F(x),"describes the probability that a random variableX with a given probability distribution will be found at a value less than or equal to x".
The exponential distribution is "the probability distribution of the time between events in a Poisson process (a process in which events occur continuously and independently at a constant average rate). It is a particular case of the gamma distribution".
Part a
Let X the random variable of interest. We know on this case that

And we know the probability denisty function for x given by:
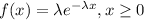
In order to find the cdf we need to do the following integral:
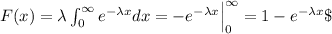
Part b
Assuming that
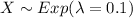 , then the density function is given by:
, then the density function is given by:

And for this case we want this probability:

And evaluating the integral we got: