Answer:
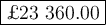
Explanation:
The formula for the accrued amount from compound interest is
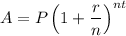
1. Amount in account on 1 Jan 2015
(a) Data:
a = £23 517.60
r = 2.5 %
n = 1
t = 1 yr
(b) Calculations:
r = 0.025
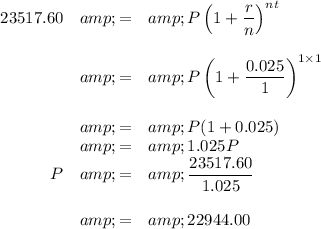
The amount that gathered interest was £22 944.00 but, before the interest started accruing, Carol had withdrawn £1000 from the account.
She must have had £23 944 in her account on 1 Jan 2015.
(2) Amount originally invested
(a) Data
A = £23 944.00

3. Summary
1 Jan 2014 P = £23 360.00
1 Jan 2015 A = 23 944.00
Withdrawal = -1 000.00
P = 22 944.00
1 Jan 2016 A = £23 517.60