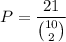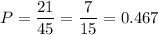Answer:
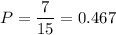
Explanation:
Probabilities
When we choose from two different sets to form a new set of n elements, we use the so-called hypergeometric distribution. We'll use an easier and more simple approach by the use of logic.
We have 6 republicans and 4 democrats applying for two positions. Let's call R to a republican member and D to a democrat member. There are three possibilities to choose two people from the two sets: DD, DR, RR. Both republicans, both democrats and one of each. We are asked to compute the probability of both being from the same party, i.e. the probability is
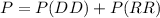
Let's compute P(DD). Both democrats come from the 4 members available and it can be done in
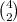 different ways.
different ways.
For P(RR) we proceed in a similar way to get
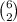 different ways.
different ways.
The total ways to select both from the same party is
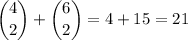
The selection can be done from the whole set of candidates in
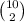 different ways, so
different ways, so