Question

Find all real zeros of the polynomials and determine the multiplicity of each. (solve by factoring)

f(x) = x^3 - 6x^2 - 9x + 54



f(x) = x^4 + 5x^3 + 6x^2

Answer 1

1) x= -3, 3, 6

2) x= -3, -2, 0

Explanation:

`f(x)=x^3-6x^2-9x+54`

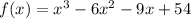
To factor this, we can use grouping

First we can factor the first pair and second pairs of terms

`x^2(x-6)-9(x-6)`

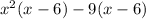
As we have the same factor on each of these, we can combine the like terms to get

`x^2-9(x-6)`

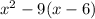
This can be factored into

`(x+3)(x-3)(x-6)`

This gives us the zeroes of

x= -3, 3, 6

`f(x)=x^4+5x^3+6x^2`

To factor this one, we first need to factor out a term

`x^2(x^2+5x+6)`

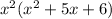
This is a quadratic equation that simplifies to

`(x^2)(x+2)(x+3)`

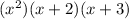
This gives the zeroes of

x= -3, -2, 0