Question

When 70.4 g of benzamide (C7H7NO) are dissolved in 850. g of a certain mystery liquid X , the freezing point of the solution is 2.7°C lower than the freezing point of pure X. On the other hand, when 70.4 g of ammonium chloride (NH4CI) are dissolved in the same mass of X, the freezing point of the solution is 9.9 °C lower than the freezing point of pure X.

A) Calculate the van't Hoff factor for ammonium chloride in X. Be sure your answer has a unit symbol, if necessary, and round your answer to 2 significant digits.

Answer 1

i=1.62 .

Step-by-step explanation:

Let, i be the Van't Hoff Factor.

Moles of benzamide,=
`(Mass)/(molar\ mass)=(70.4)/(121.14)=0.58\ mol.`

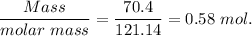
Molality of solution, m=
`(moles  )/(mass\ of\ solvent)=(0.58)/(0.85)=0.68\ molal.`

Now, we know

Depression in freezing point,
`\Delta T=i* K_f* m` .....1

It is given that,

`\Delta T=2.7^o C\\i=1 ( since\ it\ is\ non\ dissociable\ solutes)\\K_f  ( freezing\ constant)\\`

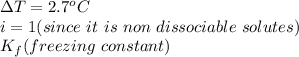
Putting all these values we get,

`K_f=3.949\ C/m.`

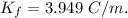
Now, moles of ammonium chloride=
`(70.4)/(53.49)=1.316\ mol.`

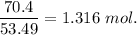
molality =
`(1.316)/(0.85)=1.54 molal.\\\Delta T=9.9 .`

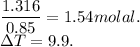
Putting all these values in eqn 1.

We get,

i=1.62 .

Hence, this is the required solution.