Question

Given sec theta=-4/3 and 90 degrees < theta< 180 degrees; find sin 2 theta

Answer 1

A

Explanation:

Answer 2

Answer is option "a" i.e.

`(-3√(7))/(8)`

Explanation:

sin2Ф = 2sinФ.cosФ

So, we need values of sinФ and cosФ but we are given secФ. We can find sinФ and cosФ with the help of secФ first by finding all sides of triangle and then by using Pythagorean theorem.

Given that,

secФ = -4/3

While,

secФ = hypotenuse/base

Hence,

length of hypotenuse = 4

length of base = 3

To find perpendicular we'll use Pythagorean theorem:

(hyp)² = (base)² + (perp)²

`perp = √(hyp^2 - base^2)`

`perp = √(16-9)\\ perp = √(7)`

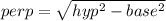
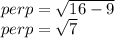
length of perpendicular = √7

Now, to find sinФ and cosФ

sinФ = perp/hyp

sinФ = √7/4

cosФ = base/hyp

cosФ = 3/4

Finally to find sin2Ф

sin2Ф = 2sinФ.cosФ

`=2*(√(7))/(4) *(3)/(4)\\=(3√(7))/(8)`

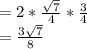
negative sign of sin2Ф

As 90≤Ф≤180

So multiplying it by 2

180≤Ф≤360

which is 3rd and 4th coordinate in which sin has negative value.