Question

1) Let f(x)=6x+6/x. Find the open intervals on which f is increasing (decreasing). Then determine the x-coordinates of all relative maxima (minima).

Let f(x)=6−4/x+2/x^2. Find the open intervals on which f is increasing (decreasing). Then determine the x-coordinates of all relative maxima (minima).



Let f(x)=(8x^2)/(x−4). Find the open intervals on which f is increasing (decreasing). Then determine the x-coordinates of all relative maxima (minima).



Let f(x)=6(x−2)^(2/3) +4. Find the open intervals on which f is increasing (decreasing). Then determine the x-coordinates of all relative maxima (minima).



Let f(x)=8√x −6x for x>0. Find the open intervals on which f is increasing (decreasing). Then determine the x-coordinates of all relative maxima (minima).

Answer 1

1) increasing on (-∞,-1] ∪ [1,∞), decreasing on [-1,0) ∪ (0,1]

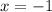`x = -1` is local maximum,
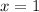`x = 1` is local minimum

2) increasing on [1,∞), decreasing on (-∞,0) ∪ (0,1]

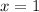`x = 1` is absolute minimum

3) increasing on (-∞,0] ∪ [8,∞), decreasing on [0,4) ∪ (4,8]

`x = 0` is local maximum,
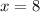`x = 8` is local minimum

4) increasing on [2,∞), decreasing on (-∞,2]

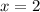`x = 2` is absolute minimum

5) increasing on the interval (0,4/9], decreasing on the interval [4/9,∞)

`x = 0` is local minimum,
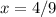`x = 4/9` is absolute maximum

Explanation:

To find minima and maxima the of the function, we must take the derivative and equalize it to zero to find the roots.

1)
`f(x) = 6x + 6/x`

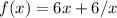
`f\prime(x) = 6 - 6/x^2 = 0` and
`x \\eq 0`

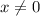
So, the roots are
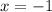`x = -1` and
`x = 1`

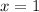
The function is increasing on the interval (-∞,-1] ∪ [1,∞)

The function is decreasing on the interval [-1,0) ∪ (0,1]

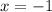`x = -1` is local maximum,
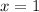`x = 1` is local minimum.

2)
`f(x)=6-4/x+2/x^2`

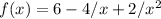
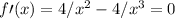`f\prime(x)=4/x^2-4/x^3=0` and
`x \\eq 0`

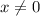
So the root is
`x = 1`

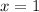
The function is increasing on the interval [1,∞)

The function is decreasing on the interval (-∞,0) ∪ (0,1]

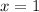`x = 1` is absolute minimum.

3)
`f(x) = 8x^2/(x-4)`

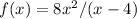
`f\prime(x) = (8x^2-64x)/(x-4)^2=0` and
`x \\eq 4`

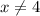
So the roots are
`x = 0` and
`x = 8`

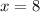
The function is increasing on the interval (-∞,0] ∪ [8,∞)

The function is decreasing on the interval [0,4) ∪ (4,8]

`x = 0` is local maximum,
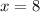`x = 8` is local minimum.

4)
`f(x)=6(x-2)^(2/3) +4=0`

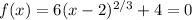
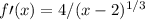`f\prime(x) = 4/(x-2)^(1/3)` has no solution and
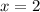`x = 2` is crtitical point.

The function is increasing on the interval [2,∞)

The function is decreasing on the interval (-∞,2]

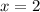`x = 2` is absolute minimum.

5)
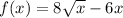`f(x)=8\sqrt x - 6x` for
`x>0`

`f\prime(x) = (4/\sqrt x)-6 = 0`

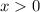
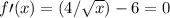
So the root is
`x = 4/9`

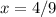
The function is increasing on the interval (0,4/9]

The function is decreasing on the interval [4/9,∞)

`x = 0` is local minimum,
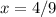`x = 4/9` is absolute maximum.