Answer:
a) U= -8.43 10 9 J b) 6.52 10⁹ J
Step-by-step explanation:
a) The potential energy can be determined from the relationship between force and energy
F = - dU / dr
dU = - F dr
We replace and integrate. If we take the positive direction in the direction of dr, the force goes in the opposite direction (attraction) therefore it is negative
F = - G mM / r²
∫ U = G mM ∫ dr / r²
U = G mM (- 1 / r)
We evaluate
 –U₀ = - G mM (1 /
–U₀ = - G mM (1 /
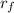 - 1 / r₀)
- 1 / r₀)
The reference system location is arbitrary, the most common choice is U₀ = 0 for r₀ = ∞
U = - G m M 1 / r
Let's reduce the magnitudes to the SI system
T = 702 min (60s / 1min) = 42120
R = 20100 km (1000m / 1km) = 2.01 10⁷ m
Let's calculate
U = -6.67 10⁻¹¹ 425 5.98 10²⁴ 1 / 2.01 10⁷
U= -8.43 10 9 J
b) The energy to place the satellite in orbit, This is equal to the mechanical energy of the system
Em = K + U
Em = ½ m v² +U
Since the satellite is in a circular orbit, the velocity module is constant
v = d / t
The distance of an orbit is the length of the circle and this time is called a period
d = 2π r
v = 2π r / T
We replace
Em = ½ m (2π r / T)² + U
Em = 2π² m r² / T² + U
Let's calculate
Em = 2π² 425 (2.01 10⁷)² / (4.2120 10⁴)² - 8.43 10⁹
Em = 1,910 10⁹ - 8.43 10⁹
Em = - 6.52 10⁹ J