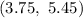Answer:
Explanation:
Given : Sample size of children : n= 20
Degree of freedom = df =n-1 =20-1=19
Sample mean years of piano lessons :
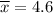
Sample standard deviation :
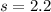
Confidence level :
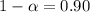
Significance level :
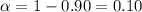
Since population standard deviation is unavailable, then
Confidence interval for the population mean :
 (1)
(1)
Using t-distribution table , we have
Critical value =
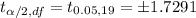
The 90% confidence interval for the average number of years students take piano lessons in this city will be :
 (Substitute the values in (1))
(Substitute the values in (1))
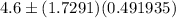
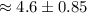

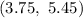
Hence, the 90% confidence interval for the average number of years students take piano lessons in this city =